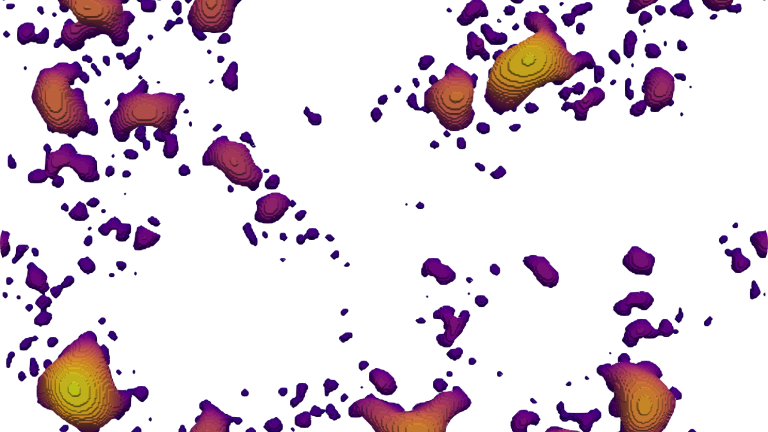
Contact between rough surfaces
A major challenge in contact mechanics stems from the multi-scale nature of phenomena occurring at the contact. Natural surfaces are made of a vast number of asperities over a wide range of length scales, which induces a much smaller contact area than the observable one.
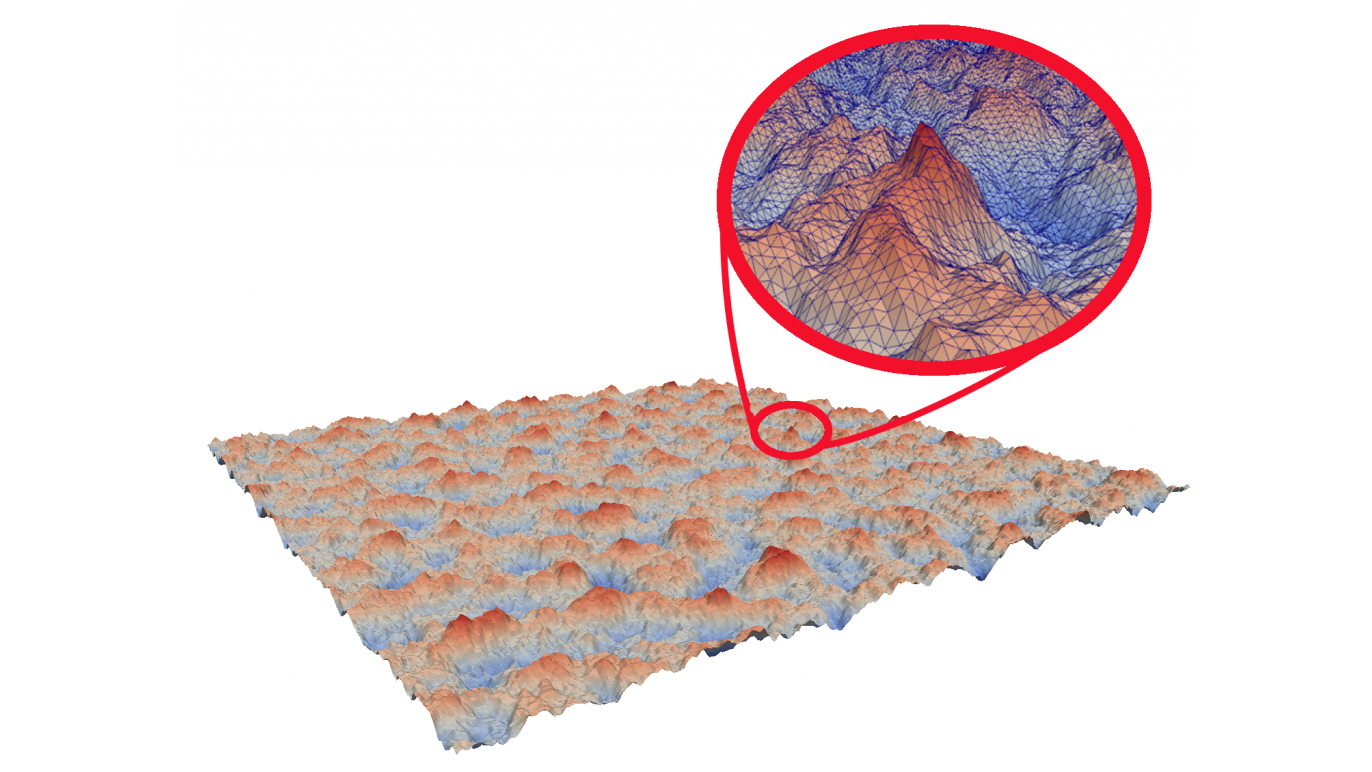
Rough surfaces are usually modeled as a Gaussian random field with a self-affine fractal-like structure. From a given rough surface, we can compute the solution of the contact problem using discretization methods such as Finite Element Method (FEM) or Boundary Element Method (BEM). In order to agglomerate sufficient statistical data, a lot of simulations have to be realized. Our research aims at developing new numerical techniques by casting the contact problem into a fully statistical framework, which will provide means to analyze statistical properties at lower costs. We collaborate with CSQI laboratory in EPFL to propose new efficient methods for the statistical analysis of contact quantities.
Recent publications
2025
Disk harmonics for analysing curved and flat self-affine rough surfaces and the topological reconstruction of open surfaces
Journal of Computational Physics
2025
Vol. 522 , p. 113578.DOI : 10.1016/j.jcp.2024.113578
2024
Two-scale concurrent simulations for crack propagation using FEM–DEM bridging coupling
Computational Particle Mechanics
2024
Vol. 11 , num. 5, p. 2235 – 2243.DOI : 10.1007/s40571-024-00788-x
2022
The INTERNODES method for applications in contact mechanics and dedicated preconditioning techniques
Computers & Mathematics With Applications
2022
Vol. 127 , p. 48 – 64.DOI : 10.1016/j.camwa.2022.09.019
The role of the roughness spectral breadth in elastic contact of rough surfaces (vol 107, pg 469, 2017)
Journal Of The Mechanics And Physics Of Solids
2022
Vol. 158 , p. 104269.DOI : 10.1016/j.jmps.2020.104269
2021
Numerical Generation and Contact Analysis of Rough Surfaces in Concrete
Journal Of Advanced Concrete Technology
2021
Vol. 19 , num. 7, p. 864 – 885.DOI : 10.3151/jact.19.864
Coupling between cohesive element method and node‐to‐segment contact algorithm: Implementation and application
International Journal for Numerical Methods in Engineering
2021
Vol. 122 , num. 16, p. 4333 – 4353.DOI : 10.1002/nme.6705
2020
Tamaas: a library for elastic-plastic contact of periodic rough surfaces
Journal of Open Source Software
2020
Vol. 5 , num. 51, p. 2121.DOI : 10.21105/joss.02121
Crack Nucleation in the Adhesive Wear of an Elastic-Plastic Half-Space
Journal Of The Mechanics And Physics Of Solids
2020
Vol. 145 , p. 104100.DOI : 10.1016/j.jmps.2020.104100
2019
Review of fundamental assumptions of the Two-Phase model for aggregate interlocking in cracked concrete using numerical methods and experimental evidence
Cement and Concrete Research
2019
Vol. 125 , p. 105855.DOI : 10.1016/j.cemconres.2019.105855
A Fourier-accelerated volume integral method for elastoplastic contact
Computer Methods in Applied Mechanics and Engineering
2019
Vol. 351 , p. 951 – 976.DOI : 10.1016/j.cma.2019.04.006
2017
The role of the roughness spectral breadth in elastic contact of rough surfaces
Journal of the Mechanics and Physics of Solids
2017
Vol. 107 , p. 469 – 493.DOI : 10.1016/j.jmps.2017.07.016
On the accurate computation of the true contact-area in mechanical contact of random rough surfaces
Tribology International
2017
Vol. 114 , p. 161 – 171.DOI : 10.1016/j.triboint.2017.04.023
Normal adhesive contact on rough surfaces: efficient algorithm for FFT-based BEM resolution
Computational Mechanics
2017
Vol. 60 , num. 1, p. 69 – 81.DOI : 10.1007/s00466-017-1392-5
2015
From infinitesimal to full contact between rough surfaces: Evolution of the contact area
International Journal Of Solids And Structures
2015
Vol. 52 , p. 83 – 102.DOI : 10.1016/j.ijsolstr.2014.09.019
2014
Impact of internal crystalline boundaries on lattice thermal conductivity: Importance of boundary structure and spacing
Applied Physics Letters
2014
Vol. 105 , num. 19, p. 194102.DOI : 10.1063/1.4901887
The existence of a critical length scale in regularised friction
Journal of the Mechanics and Physics of Solids
2014
Vol. 63 , p. 40 – 50.DOI : 10.1016/j.jmps.2013.10.007
The Contact of Elastic Regular Wavy Surfaces Revisited
Tribology Letters
2014
Vol. 56 , num. 1, p. 171 – 183.DOI : 10.1007/s11249-014-0395-z
2013
A molecular dynamics and finite element study of the nanoscale thermal contact conductance
International Journal of Heat and Mass Transfer
2013
Vol. 59 , p. 384 – 392.DOI : 10.1016/j.ijheatmasstransfer.2012.12.003
2012
The effect of loading on surface roughness at the atomistic level
Computational Mechanics
2012
Vol. 50 , num. 3, p. 273 – 283.DOI : 10.1007/s00466-011-0574-9
Contact between representative rough surfaces
Physical Review E
2012
Vol. 86 , num. 3, p. 035601.DOI : 10.1103/PhysRevE.86.035601