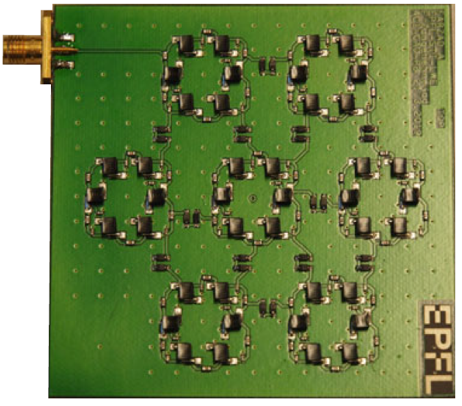
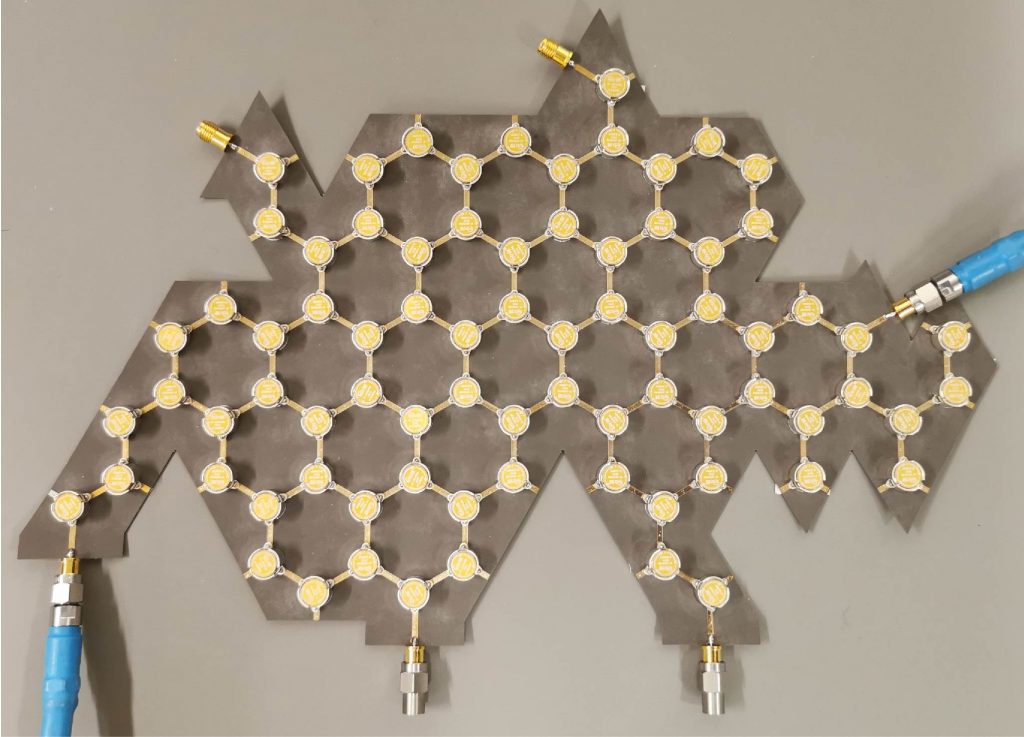
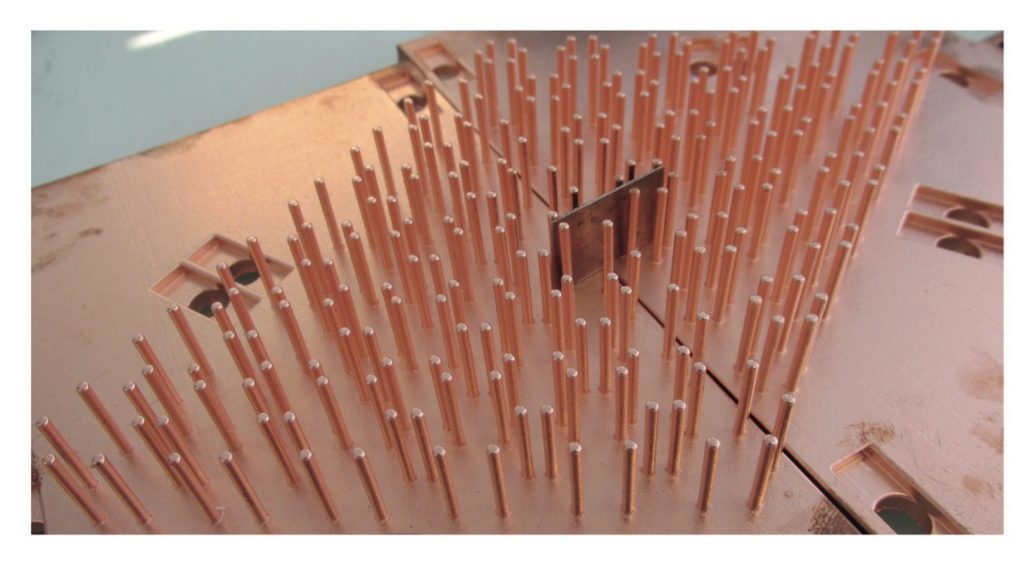
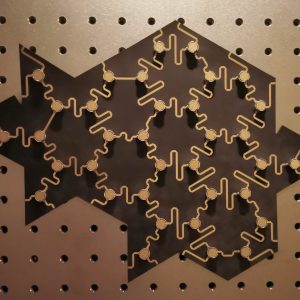
Introduction
Topology, the branch of mathematics that studies continuous transformations, can have very concrete and powerful consequences on physical systems. Topology allows to define global invariants, that do not change upon continuous deformations, such as geometrical changes, the presence of impurities, or distributed imperfections coming from fabrication tolerances. When applied to wave phenomena, topology can guarantee that a given property of the wave, for example its propagation along a given path or its scattering signature, is protected against different kinds of perturbations, imperfections or reconfigurability.
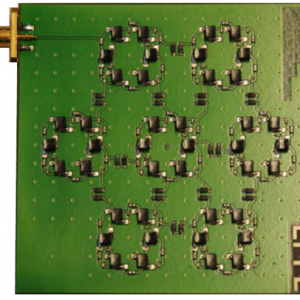
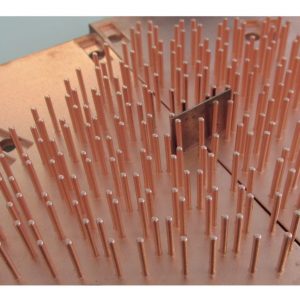
At the laboratory of Wave Engineering, we research the applications of topology to the design of simpler, smaller, more robust, or more flexible microwave, acoustic and fluid devices .
To learn more
- Z. Zhang, P. Delplace and R. Fleury, “Anomalous nonreciprocal topological networks: stronger than Chern insulators“, Nature, 2021.
- R. Fleury, “The sound of Weyl hinges“, Nature Materials, 2021.
- C. Coulais, R. Fleury and J. Van Wezel, “Topology and broken Hermiticity“, Nature Physics, 2021.
- F. Zangeneh-Nejad and R. Fleury, “Zero-index Weyl metamaterials“, Physical Review Letters, 2020.
- F. Zangeneh-Nejad and R. Fleury, “Disorder-induced signal filtering with topological metamaterials“, Advanced Materials, 2020.
- F. Zangeneh-Nejad and R. Fleury, “Topological wave insulators: A review“, Comptes Rendus Physique, 2020.
- F. Zangeneh-Nejad and R. Fleury, “Non-linear second order topological insulators“, Physical Review Letters, 2019. (cover paper)
- B. Orazbayev and R. Fleury, “Quantitative robustness analysis of topological edge modes in C6 and valley-Hall metamaterial waveduides“, Nanophotonics, 2019.
- F. Zangeneh-Nejad and R. Fleury, “Topological analog signal processing“, Nature Communications, 2019.
- F. Zangeneh-Nejad and R. Fleury, “Topological Fano resonances“, Physical Review Letters, 2019.
- S. Yves, R. Fleury, T. Berthelot, M. Fink, F. Lemoult and G. Lerosey, “Crystalline metamaterials for topological properties at subwavelength scale“, Nature Communications, 2017.
- S. Yves, R. Fleury, F. Lemoult, M. Fink and G. Lerosey, “Topological acoustic polaritons: robust sound manipulation at the subwavelength scale“, New Journal of Physics, 2017.
- R. Fleury, A.B. Khanikaev and A. Alu, “Floquet topological insulators for sound“, Nature communications, 2016.
- A.B. Khanikaev, R. Fleury, S. H. Mousavi and A. Alu, “Topologically robust sound propagation in an angular-momentum-biased graphene-like resonator lattice“, Nature communications, 2016.
Involved LWE researchers
Junda Wang, Haoye Qin (Ph.D. students)
Zhe Zhang, Benjamin Apffel, Qiaolu Chen (Postdoc)
Collaborations
Pierre Delplace, ENS de Lyon